



Next: The degree of the
Up: The theory of permutations
Previous: The theory of permutations
Albert Girard (1590-1633), a flemish mathematician, published in 1629, in
Amsterdam, a book called Invention nouvelle en l'algèbre, in which
clear relations between roots and coefficients of polynomials were stated
for the first time. Let 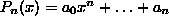 where
where  .
Girard had the brilliant idea of postulating that such a polynomial had
to have n roots; whenever a polynomial had less than n real roots, one could
extend the set of the roots to have order n by adjoining complex
roots
.
Girard had the brilliant idea of postulating that such a polynomial had
to have n roots; whenever a polynomial had less than n real roots, one could
extend the set of the roots to have order n by adjoining complex
roots . Under these conditions, one could write
. Under these conditions, one could write
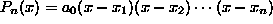 where
where 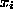 is the
is the 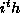 root. Hence
the derivative is given by
root. Hence
the derivative is given by 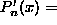
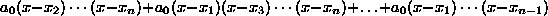
hence

Notice that for each 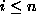 we have
we have

Now, provided 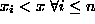 , we have
, we have
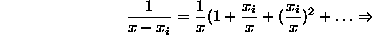

Now let 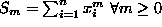 . This means
. This means

which gives infinite relations between coefficients and roots. Take for
example 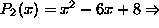
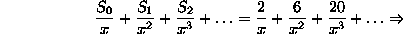
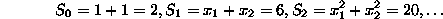
Although it is possible to derive infinitely many such relations, to isolate
one single root one needs at least a difference between some powers of the
roots. In the case of the quadratic  we have
we have

hence we can isolate the roots and solve the equation.
Leo Liberti
Thu Feb 26 17:04:11 CET 1998